Invariant Submanifold of Ψ(4K+3,1) Structure Manifold

Authors

  • Lakhan Singh  Department of Mathematics, D. J. College, Baraut, Baghpat, Uttar Pradesh, India
  • Shailendra Kumar Gautam  Eshan College of Engineering, Mathura, Uttar Pradesh, India

Keywords:

Invariant Submanifold, Nijenhuis Tensor, Projection Operators And Complementary Distributions.

Abstract

In this paper, we have studied various properties of a 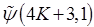structure manifold and its invariant sub manifold, where K is a positive integer greater than or equal to one. Under two different assumptions, the nature of induced structure , has also been discussed.

References

  1. A Bejancu: On semi-invariant submanifolds of an almost contact metric manifold. An Stiint Univ., "A.I.I. Cuza" Lasi Sec. Ia Mat. (Supplement) 1981, 17-21.
  2. B. Prasad: Semi-invariant submanifolds of a Lorentzian Para-sasakian manifold, Bull Malaysian Math. Soc. (Second Series) 21 (1988), 21-26.
  3. F. Careres: Linear invairant of Riemannian product manifold, Math Proc. Cambridge Phil. Soc. 91 (1982), 99-106.
  4. Endo Hiroshi: On invariant submanifolds of connect metric manifolds, Indian J. Pure Appl. Math 22 (6) (June-1991), 449-453.
  5. H.B. Pandey & A. Kumar:Anti-invariant submanifold of almost para contact manifold. Prog. of Maths Volume 21(1): 1987.
  6. K. Yano:On a structure defined by a tensor field f of the type (1,1) satisfying f3+f=0. Tensor N.S., 14 (1963), 99-109.
  7. R. Nivas & S. Yadav:On CR-structures and - HSU - structure satisfying , Acta Ciencia Indica, Vol. XXXVII M, No. 4, 645 (2012).

International Journal of Scientific Research in Science, Engineering and Technology

Downloads

Published

2015-12-25

Issue

Volume 1, Issue 6, November-December-2015

Section

Research Articles

License

Copyright (c) IJSRSET

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

How to Cite

[1]
Lakhan Singh, Shailendra Kumar Gautam "Invariant Submanifold of Ψ(4K+3,1) Structure Manifold " International Journal of Scientific Research in Science, Engineering and Technology (IJSRSET), Print ISSN : 2395-1990, Online ISSN : 2394-4099, Volume 1, Issue 6, pp.306-309, November-December-2015.